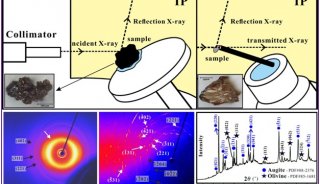
Low Loss Sapphire Windows for High Power Microwave Transmission(五)
Single crystal sapphire is not a typical optical material, with a standard strength not much lower than the steel described above, since the average failure strength for sapphire with a standard polish is approximately 420 MPa.
For a sapphire disk (E = 345 x 109 Pa) under similar conditions as that specified above for a steel disk, a sapphire disk would be 1.2 mm thick assuming linear theory. The stress factor would be about 2, which is on the borderline of the large deflection regime.
The fact that standard sapphire mounted in the usual manner is on the borderline of the large deflection regime, is critical to the effort described in this report to create very thin sapphire microwave windows. For most materials failure strength is a fixed, prespecified property of a given material. For sapphire the failure strength varies by a large amount as a result of both the statistical nature of the failure of sapphire as a ceramic, and as a result of polish strengthening. By using polishing strengthening and appropriate edge mounting techniques, sapphire windows can be designed to function well into the large deflection regime, so that linear theory greatly overestimates the necessary disk thickness. The disks can be made much thinner because stresses are distributed throughout the window by membrane forces.
When membrane forces are significant, the response of a disk to loading is fundamentally changed, as is disk design. Linear theory predicts that the design thickness of the disk depends on the square root of the loading, which is a weak dependence. For linear theory, a factor of 2 reduction in load, which is a major decrease, only allows a factor of 1.4 reduction in disk thickness. In the large deflection regime, an increase in failure strength by a factor of 2 (by polish strengthening, for instance) can result in a factor of 3 thinner disk, which is a major reduction in terms of microwave absorption. The influence of membrane effects may modify the degree to which sapphire can be polish strengthened, however, since this strengthening relies on the fact that surface flaws limit the inherent strength of sapphire. When the peak stress in the sapphire is more uniformly distributed throughout the thickness of the disk rather than at a surface, polish strengthening may be less important.
Another peculiarity of the present effort is the degree to which failure strength and edge boundary conditions affect the engineering behavior of the disks. In most cases, for relatively thick disks the central stress is not dependent on edge conditions because the deflection at the edge of a strong disk is negligible. Edge boundary conditions are well known for their effect on the stress in mechanical systems, and these effects become important for very thin sapphire disks where edge deflections become significant under load.
Quantitative Prediction. Accurate theoretical prediction of the stress and deflection for the real case of the cantilevered sapphire window is not possible analytically, but must be done computationally. The primary engineering goal of modeling is to predict the failure stress to an accuracy of 10 to 20%. As an approximation to large deflection theory [15] the deflection w0 at the center of the disk is given by the equation:
w0/h + A(w0/h)3 = B(q/E)(a/h)4
where w0 is the (maximum) deflection at the center, and A and B are constants determined by the boundary conditions on the disk. The constants that are given in Timoshenko for this equation, and used for the calculations presented below assume a Poisson's ratio, ν = 0.3, rather than ν = 0.25 for sapphire, but the corrections for this difference should be small. There are four fundamental edge conditions given:
1) Plate clamped, edge immovable.
2) Plate clamped, edge free.
3) Plate simply supported, edge immovable.
4) Plate simply supported, edge free.
These different conditions lead to very different predictions of the stress in the disk, as in the case of linear theory. Clamping the edge provides substantial moment at the edge of the disk when it is loaded. Making the edge immovable forces the disk to stretch as it deflects, increasing membrane effects.
Figure 7 shows linear theory and large deflection theory predictions for a 1 mm thick sapphire disk of approximately 100 mm in diameter. The solid straight line bounding all of the curves at the top is the linear prediction of stress versus pressure loading. The two large deflection theory, simply supported boundary condition cases diverge from this linear theory near 1 atm of pressure. Note how well these calculations agree with the estimations about how close this size sapphire disk would be to the large deflection regime at this pressure. For both clamped boundary conditions the linear and large deflection theories do not diverge very much at the pressures shown. The linear theory for the clamped boundary conditions is not shown; it is a straight-line tangent to the low pressure data for the large deflection theory using this boundary condition.

Figure 7. Large deflection and linear theory predictions for a sapphire disk with thickness 1.0 mm and radius 44.6 mm mounted at its edge.
Assuming standard sapphire is used with a failure stress of 420 MPa, a sapphire disk of these parameters would fail at 1.75 atm according to linear theory, but in reality it would fail at just over 3 atm for the simply supported, edge immovable case, a 70% increase in loading. Strengthened sapphire would result in even larger loading differences between the linear and large deflection predictions. For only a 50% strengthening of the sapphire the failure load would increase from 2.5 atm for linear theory to 5.5 atm in the large deflection, simply supported, edge immovable case. This is a 120% increase in load carrying capacity, a large factor. The difference increases as the disk thickness is decreased to minimize the thickness for failure at a specific loading, so that the disk behavior is farther into the membrane regime.
It can be seen that the edge free and edge immovable boundary conditions lead to very different predictions in the large deflection theory. This is because constraining the edge radially forces the disk to stretch, and this effect enhances membrane effects. In many practical cases an immovable edge is a better approximation to experimental conditions than is a free edge because the disk extends well beyond the aperture. The sapphire material beyond the aperture acts to constrain the material at the aperture edge to so that it cannot move radially. Any kind of brazing at the edge of the disk also tends to make the edge boundary condition closer to an immovable edge.
Cantilevering the disk by making it significantly larger than the aperture and sealing the outer edge tends to approximate the clamped edge boundary condition. This technique uses pressure on the disk area that is outside the aperture to counterbalance the pressure inside the aperture. The counterbalancing forces are transmitted to the center of the disk by moments at the disk aperture, which is exactly the boundary condition of a clamped edge. A clamped edge is a special case of cantilevering where the pressure on the outer part of the disk is sufficient to keep the disk flat against the support at a radius equal to and larger than the aperture radius. Less pressure allows the disk to lift off of the surface, so that the actual boundary condition for a cantilevered disk is somewhere between the clamped and simply supported case. The effect of cantilevering relative to the case of a simply supported disk can be assessed by examining the case of the clamped boundary condition for a disk with the same aperture. The larger and thinner the disk is, the better will be the approximation to the clamped boundary condition. It is also important to note that just as the cantilevering does not provide enough force to provide true clamping, it also reduces the stress at the aperture edge, which is very high for the fully clamped case. In the fully clamped case the stress is a maximum at the edge of the disk.
Window Testing/Modeling Comparison. The above discussion presents a complex description of the behavior of a thin sapphire disk where it is difficult to predict failure stress from failure pressure and where it is seen to be difficult to predict the precise boundary conditions in a real experiment. This complexity makes analysis difficult but is appropriate for practical use, none the less. The description of an aperture-mounted disk with varying edge boundary conditions arises from what is the most common and simple experimental/industrial practice for mounting windows.
Testing Apparatus. Hydraulic pressure tests were done to measure window performance and to mimic the actual use conditions of a microwave window. Pressure testing to failure is straightforward to do. The pressure gauges used to monitor failure pressure are accurate to a few percent. The mounting configuration is an industry and commercial standard. Translation of the tests to commercial practice are direct within the statistical failure of the sapphire. Correlating experimental pressure testing results with the standard characterization of sapphire is much more complex. The standard failure strength of sapphire is generally given as a minimum tensile strength with considerable statistical variation. The maximum tensile stress in a disk during a pressure test is difficult to derive from modeling in the presence of membrane effects and mixed boundary conditions.
A hydraulic pressure failure testing facility shown in Fig. 8 was used to test the behavior of sapphire windows in response to pressure loading for this program. The fixture consists of two conflat flanges bolted together and sealed by knife edges in the flanges that simultaneously deform a copper gasket. Pressure is supplied by a medium pressure hydraulic hand pump. Two 2% accuracy pressure gauges with ranges from 0-0.4 MPa, and 0-2.8 MPa, measured pressure that was recorded with a computer data acquisition system. The windows are sealed to the flanges using an O-ring in an appropriately sized groove. A segmented O-ring placed between the upper flange and the window is used to force the window against the O-ring for initial sealing before pressurization.

Figure 8. Schematic of hydraulic pressure failure testing fixture and deflection measuring apparatus.
Different size windows can be tested in the same fixture by fabricating a flange with stepped recesses with different size O-ring grooves as shown in Fig. 9. A total of three separate fixtures were used to test windows ranging from 12.5 mm to 100 mm in diameter. For the largest windows the central deflection measurements of the

Figure 9. Sapphire disk pressure testing flange.
-
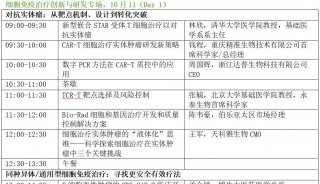
政策法规

-
政策法规

-
科技前沿

-
会议会展

-
焦点事件

-
焦点事件

-
焦点事件

-
焦点事件

-
会议会展

-
焦点事件

-
招标采购

-
焦点事件
-
焦点事件

-
焦点事件

-
会议会展

-
焦点事件

-
企业风采

-
焦点事件

-
焦点事件

-
项目成果

-
焦点事件

-
焦点事件

-
焦点事件

-
焦点事件
-
焦点事件

-
焦点事件

-
焦点事件

-
焦点事件

-
焦点事件

-
焦点事件

-
焦点事件

-
焦点事件

-
政策法规

-
焦点事件

-
焦点事件

-
政策法规

-
精英视角

-
焦点事件

-
焦点事件

-
焦点事件

-
焦点事件

-
焦点事件
-
焦点事件

-
焦点事件
-
科技前沿